Pythagoras Picture was developed on July 29, 2024.
A03. Pythagoras
First revision: Jul.29, 2024
Last change: Dec.28, 2024
Searched, Gathered, Rearranged, Translated, and Compiled by Apirak Kanchanakongkha.
1.
page 1
Number Is The Ruler Of Forms And Ideas
PYTHAGORAS
(c.570-495 BCE.)
| IN CONTEXT |
| |
BRANCH |
Metaphysics |
| |
APPROACH |
Pythagoreanism |
| |
BEFORE |
6th century BCE. Thales proposes a non-religious explanation of the cosmos. |
| |
AFTER |
c.535-c.475 BCE. Heraclitus dismisses Pythagoreanism and says that the cosmos is governed by change.
c.428 BCE. Plato introduces his concept of perfect Forms, which are revealed to the intellect and not the senses.
c.300 BCE. Euclid, a Greek mathematician, establishes the principles of geometry.
1619 - German mathematician Johannes Kepler describes the relationship between geometry and physical phenomena. |
1.
Western philosophy was still in infancy when Pythagoras was born in Miletus, Greece. A group of philosophers collectively known as the Milesian School had begun to seek rational explanations for natural phenomena only a generation earlier, marking the beginning of the Western philosophical tradition. Pythagoras spent his childhood not far from Miletus, so he likely knew them (the Milesians) and even studied in their school. Like Thales, the founder of the Milesians, Pythagoras is said to have learned the basics of geometry during his travels to Egypt. Given this background, it is unsurprising that he would have taken a scientific and mathematical approach to philosophical thought.
1.
2.
page 2
The Pythagorean Academy
However, Pythagoras was a deeply religious and superstitious man. He believed in the reincarnation and transmigration of souls and founded a religious order, representing himself as the Messiah, in Croton, southern Italy. His followers lived in a commune, following strict rules of behavior and diet while studying his religious and philosophical theories. His followers, the Pythagoreans, considered his ideas mystical revelations to the extent that some of his supposed “revelations” may have come from others in the community. His followers recorded his ideas, including Theano of Crotona, his wife, and their daughters. The two views of Pythagoras – the mystical and the scientific – seemed irreconcilable. But Pythagoras himself did not see them as contradictory. To him, The goal of life is freedom from the cycle of reincarnation, which comes from adhering to strict behavioral rules and from reflecting, or what we call objective scientific thinking, on the truths he encounters that he considers self-evident, as if given by God, and mathematically proven to have implications for God's revelation.
Pythagoras, source: medium.com, access date: Jul.29, 2024.1.
Pythagoras
Very little is known about Pythagoras' life. Pythagoras did not write anything, and unfortunately, the Greek philosopher Porphyry stated in his Vita Pythagoras that "it is not known for certain what Pythagoras told his colleagues since they observed an unusual silence." Modern scholars believe that Pythagoras may have been born on Samos, off the coast of modern Türkiye. As a young man, he traveled widely, perhaps studying at the Milesian school and possibly visiting Egypt, a center of learning at the time. When he was about 40, he founded a community of about 300 members in Croton, in southern Italy. Its members studied both mystical and academic subjects, and although the community was a group, Pythagoras was its leader. At 60, he married a young woman named Theano of Crotona. However, his growing hatred of the Pythagorean cult eventually led him to leave Croton, and he fled to Metapontum, also in southern Italy, where he died shortly afterward. His community virtually disappeared by the end of the 4th century BC.
From Facebook "The Stoicism Digest" Page, access date: Dec.10, 2024.1.
page 3
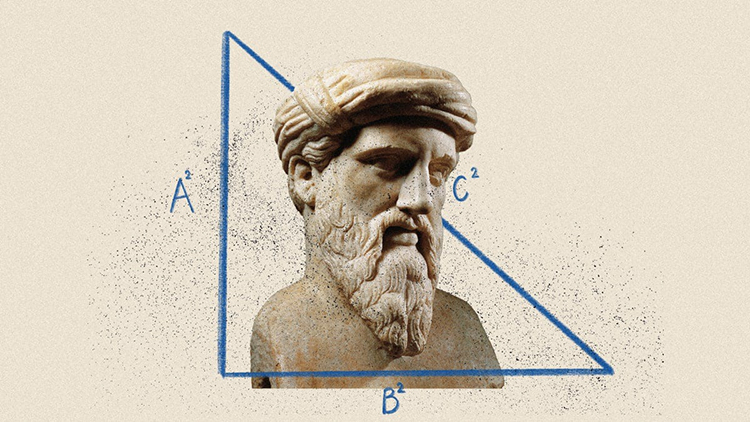
Since these mathematical discoveries were based purely on reason, Pythagoras believed they were worth more than mere observation. For example, the Egyptians discovered that a triangle with a ratio of 3:4:5 must always have a right angle, which had practical uses, such as in architecture. But Pythagoras found the basic principle behind all right triangles (that the square of the hypotenuse equals the sum of the squares of the other two sides) and found it to be a universal truth. This discovery was so extraordinary and potent that the Pythagoreans considered it a revelation from God.
Pythagoras concluded that mathematical laws must govern the entire universe. He said that numbers (numerical ratios and mathematical axioms) could explain the universe's structure. He accepted the Milesian idea that the universe consisted of a single basic substance but changed the inquiry from substance to form.
This was such a radical change in our view of the world that we should forgive Pythagoras and his followers for indulging in the mystical significance of numbers. They discovered the squares and cubes we speak of today by exploring the relationship between numbers and geometry. However, they also identified specific characteristics of these numbers, such as even as “good,” odd as “bad,” and even four as “justice.” The number ten, a tetractys (or tetractis — a triangle with a row of points) had special significance in Pythagorean ritual. They saw the number one as a single point. It was a unity that could stand in for everything else. It was less controversial. In this conception, two was a line, three was a surface or plane, and four was a solid. The correspondence is straightforward with our current conception of dimensions.
Classical architecture uses the mathematical principle of Pythagorean ratios, using harmonious shapes and proportions throughout the structure, reducing its size and increasing its overall size. This image was developed on December 28, 2024.
1.
2.
3.
